Test your luck and see how long it takes you to escape from the Cosmic Microwave background. An engaging boardgame for communicating the complex astrophysical concept of random chance.

This activity is best suited to students in upper primary and junior secondary school. It requires a good level of concentration as there is a possibility that any given student (or team of students) may have to make many tens of rolls of the dice to finish the maze, where another student could take as little as three.
THE SCIENCE
After the Big Bang, the newly formed Universe was a dense, hot soup of simple particles. The heat meant there was lots of energy and lots of light, but because everything was squashed together the light couldn’t travel very far.
A light packet (photon) would be randomly released by an energetic particle but was almost immediately caught by another particle. Catching that photon made this new particle energetic, so it too would release a photon at random, and the cycle continued. It was not until the Universe expanded and cooled, and enough space appeared so that the light could finally travel freely through the Universe for the first time. This happened about 380,000 years after the Big Bang.
Most of that light is still travelling today. Some of it lands on our radio telescopes and is 13 billion years old. This ancient light is Cosmic Microwave Background.
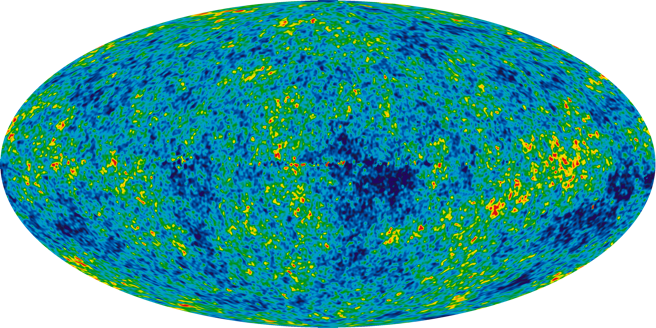
Cosmic Microwave background (NASA, Public domain, via Wikipedia Commons)
THE ACTIVITY
- Print out the ASTRO 3D ‘Escape the CMB maze’. Up to four students or teams can play on a single ‘board’.
- At least one 4-sided dice (one dice per team). If you do not have a dice there is a template below for printing and constructing one. The other option is to use a random number generator such as random.org
- A different coloured counter per team
- Pencils
- Graph paper or a computer to graph the results of the teams (optional).
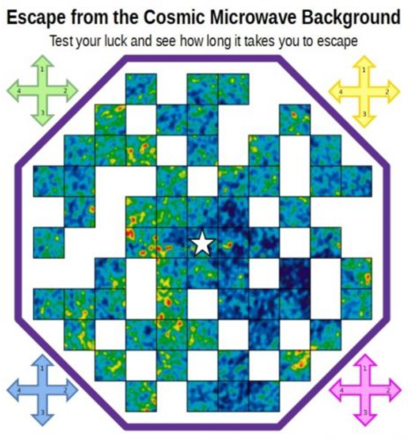
How to Play
- Start your counter on the star in the middle. Roll the dice. The number on the ‘apex’ of the dice indicates the direction you move your counter one coloured space. The direction is indicated by the arrows on the board.
- Keep a tally each time you roll the dice.
- Keep rolling the dice until you reach the purple border. Congratulations, you have escaped the early Universe!
CURRICULUM LINKS V 9.0
Year 4 -Mathematics – Probability
Conduct repeated chance experiments to observe relationships between outcomes; identify and describe the variation in results (AC9M4P02)
- Playing games….and deciding if it makes a difference who goes first and whether you can use a particular strategy to increase your chances of winning
Year 5 – Mathematics – Probability
List the possible outcomes of chance experiments involving equally likely outcomes and compare to those which are not equally likely (AC9M5P01)
- Commenting on the chance of winning games b considering the number of possible outcomes and the consequent chance of winning
- Investigating why some games are fair and others are not; for example, drawing a track game to resemble a running race and taking it in turns to roll 22 dice, where the first runner moves a square if the different between the 22 dice is zero, one or 22 and the second runner moves a square if the different is 3, 43, 4 or 55; responding to the questions, “Is this game fair?”, “Are some differences more likely to come up than others?” and “How can you work that out?”